Cosmologie masse manquante univers jumeaux

The missing mass problem (p4)

5) The large size structure of the universe

From the equation (24) we see that if a cluster exists in one fold, an associated diffuse halo structure exists in the conjugated region of the second fold. If this model is correct we should find halo-structures in our fold of the universe. With the help of Dr. Pierre Midy, from the university of Orsay, France, we have performed numerical simulations, using a Cray-1 computer. We consider two distributions of 350 points. The first is represented by little circles and the second by small crosses. At the begining the points are randomly distributed on the screen and are supposed to represent two uniform gazes. Each mass owns a random velocity corresponding to an isotropic Maxwellian distribution with an averaged thermal velocity <V> . Call m1 the elements of the first population and m2 the elements of the second population. We apply the Newton law with

m1 attracts m1 : gravitational effect

m2 attracts m2 : gravitational effect

m1 and m2 repel each other : antigravitational effect

...We consider this two-dimensional system as periodic over space. In other terms the upper boundary is linked to the lower one and the right to the left (Euclidean 2D torus). So that we can compute the sum of the mutual actions of the particles. For each interval of time ?t we compute the acceleration of each particle and determine the trajectory by Taylor expansion. Each particle that comes out through the right boundary reappears through the left one, and same thing for the upper and lower boundaries. This makes possible to study the gravitational instability of these two coupled systems in a finite portion of space (with toroidal topology). The interval of time is determined in order to get significant computational results. In other terms we demand the trajectory of a particle to be approximatively regular. The following figures show the typical behaviour of the system after 4000 intervals of time. In figures 4 and 5 we find both clusters and cellular patterns. This is enhanced in the figures 7 and 8.

Fig. 4 : Effect of the gravitational instability on the system 1

Fig. 5 : Effect of the gravitational instability on the system 2

Fig 6 (ga3127): Superposition of the two.

Fig 7.: Enhanced spatial distribution of the two populations.

Fig 8.. White : population 1. Grey : population 2

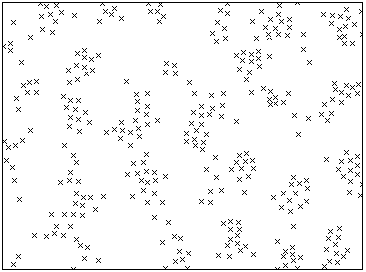
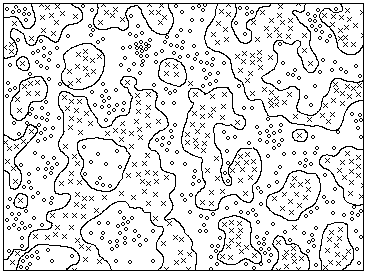
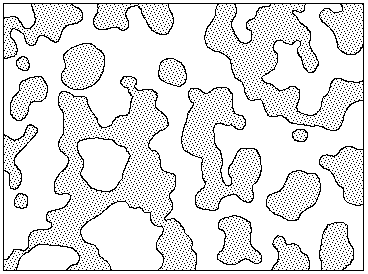
...We suggest that such a mechanism could explain the large scale structure of the universe and the observed distribution of galaxies. Suppose that our fold of the universe corresponds to the population 1. In the right lower part of the screen this matter is arranged around large "empty" bubbles. These bubbles correspond to a cluster arrangement in the population 2, supposed to be located in the second fold of the universe (in fact the antipodal region), according to our theory. But, as seen on the figure 8, for a given population, in some places the matter can be arranged as a Swiss "gruyère" cheese and in other places as an emulsion.

...These first crude numerical simulations have to be developped with a larger number of points and in a three-dimensional representation. We know that the three-dimensional's behaviour of a system can be somewhat different from the two-dimensional's one. But we expect the conclusions to be similar. We think that with a larger number of points we could get a fractal system, as suggested in the figure 14, but we precise that this peculiar computation has not yet been done, but are under study. According to this idea the galaxies should be located in the holes of the associated anti-matter cloud, which would ensure their confinement, as suggested earlier.